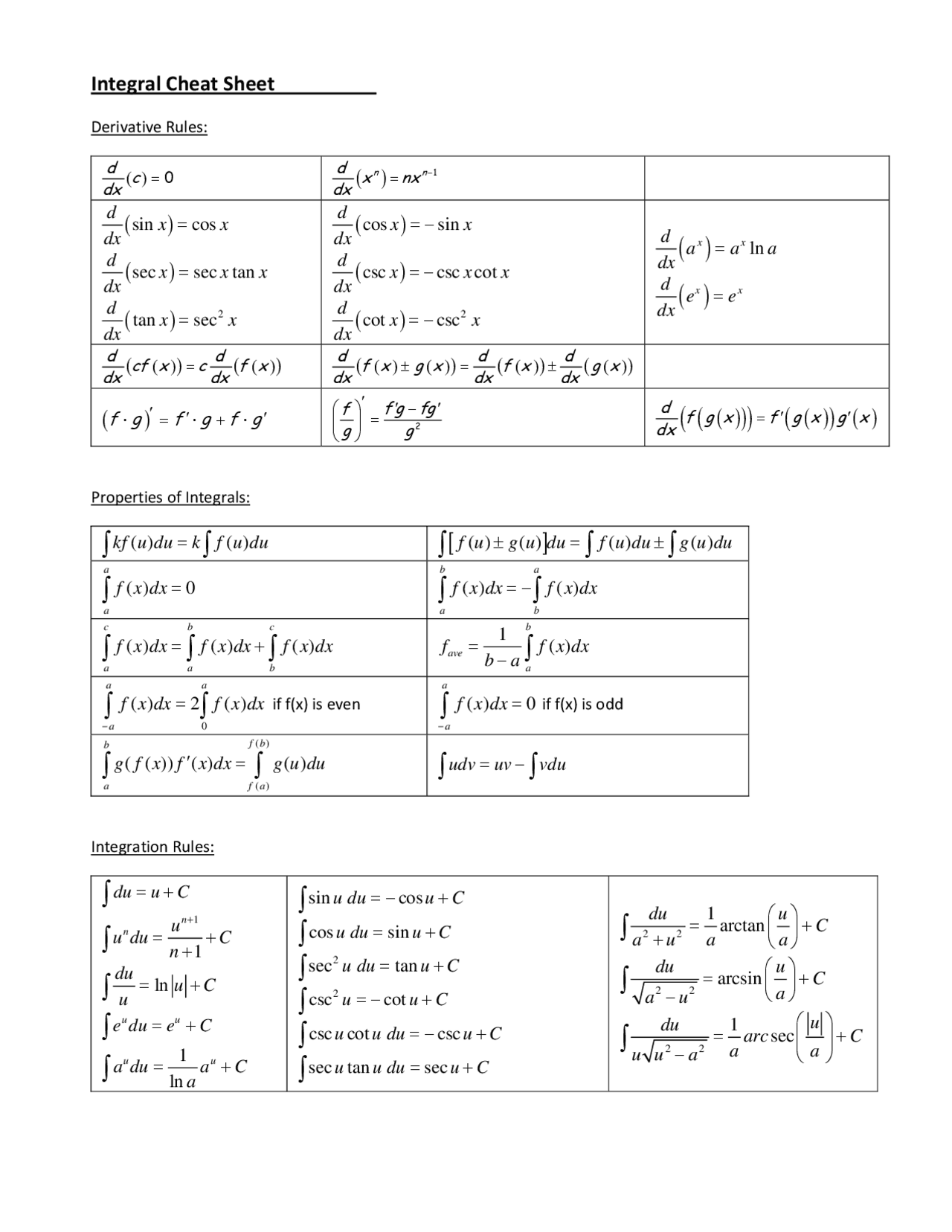
Integral Cheat Sheet: Your Ultimate Guide To Mastering Calculus
Let’s be real—integral calculus isn’t exactly a walk in the park. Whether you’re a student cramming for an exam or a professional brushing up on your math skills, having a solid integral cheat sheet can be a lifesaver. This guide is packed with all the essential formulas, tips, and tricks you need to conquer integrals like a pro. So buckle up, because we’re about to dive deep into the world of calculus!
Now, let’s talk about why you’re here. You’re probably looking for a quick and reliable way to understand integrals without losing your mind. That’s where this cheat sheet comes in. It’s like having a personal tutor in your pocket, ready to explain everything from basic integration rules to advanced techniques.
But before we get into the nitty-gritty, let’s clear something up. An integral cheat sheet isn’t just a list of formulas—it’s a tool that helps you think critically and solve problems efficiently. So whether you’re a math enthusiast or just trying to survive calculus class, this guide has got you covered.
Read also:Toca Mexican The Ultimate Spot For Authentic Mexican Flavors
What Exactly Is an Integral?
First things first—what even is an integral? Simply put, an integral is the reverse process of differentiation. Think of it as the “undo button” for derivatives. It helps you calculate areas under curves, volumes of solids, and all sorts of cool stuff in physics and engineering.
There are two main types of integrals: definite and indefinite. A definite integral gives you a specific value, while an indefinite integral gives you a family of functions. Make sense? Cool. Now let’s break it down further.
Key Components of Integrals
Here’s a quick rundown of the key components you’ll encounter:
- Integrand: The function you’re integrating.
- Variable of Integration: The variable with respect to which you’re integrating (usually x).
- Limits of Integration: The boundaries for definite integrals.
- Constant of Integration: The "+ C" you always see in indefinite integrals.
These components might sound fancy, but once you practice a bit, they’ll feel like second nature. Trust me.
Why Do You Need an Integral Cheat Sheet?
Here’s the thing—integrals can get messy. Really messy. From trigonometric functions to exponential equations, there’s a lot to keep track of. That’s where an integral cheat sheet comes in handy. It’s like a roadmap that helps you navigate the chaos of calculus.
But wait—why not just memorize everything? Well, memorization only gets you so far. A cheat sheet helps you understand the relationships between different formulas and techniques, making it easier to apply them in real-world scenarios.
Read also:Dynamic Golfer Unleashing The Power Of Modern Golf Mastery
Benefits of Using a Cheat Sheet
Here’s why you should totally use an integral cheat sheet:
- Saves Time: Instead of flipping through textbooks, you can quickly reference key formulas.
- Boosts Confidence: Knowing you have a reliable resource at your fingertips makes problem-solving less intimidating.
- Improves Accuracy: With all the formulas in one place, you’re less likely to make mistakes.
So yeah, an integral cheat sheet isn’t just a shortcut—it’s a powerful learning tool.
Basic Integration Rules
Alright, let’s get down to business. Here are some of the most fundamental integration rules you need to know:
1. Power Rule
This one’s a classic. If you’re integrating x^n, the formula is:
∫x^n dx = (x^(n+1))/(n+1) + C
Simple, right? Just increase the exponent by 1, divide by the new exponent, and don’t forget the "+ C"!
2. Constant Rule
If you’re integrating a constant, say k, the formula is:
∫k dx = kx + C
Basically, you just multiply the constant by x and add the constant of integration.
3. Sum and Difference Rule
When you’re dealing with multiple terms, you can integrate them separately:
∫(f(x) ± g(x)) dx = ∫f(x) dx ± ∫g(x) dx
This rule makes life a whole lot easier when you’re working with complex functions.
Advanced Techniques for Integration
Now that we’ve covered the basics, let’s talk about some more advanced techniques. These are the ones that’ll really set you apart as a calculus whiz.
1. Substitution Method
Also known as u-substitution, this technique is perfect for integrals that involve composite functions. The idea is to replace a complicated part of the function with a simpler variable, making it easier to integrate.
For example, if you’re integrating ∫cos(2x) dx, you can let u = 2x, so du = 2dx. Then the integral becomes:
(1/2)∫cos(u) du
See how much simpler that is?
2. Integration by Parts
This one’s a bit trickier, but super useful for products of functions. The formula is:
∫u dv = uv - ∫v du
The key is choosing u and dv wisely. A common mnemonic to help with this is “LIATE,” which stands for Logarithmic, Inverse trigonometric, Algebraic, Trigonometric, and Exponential functions.
3. Partial Fraction Decomposition
This technique is your go-to for rational functions (fractions with polynomials in the numerator and denominator). The idea is to break the fraction into simpler parts that are easier to integrate.
For example, if you’re integrating ∫(3x+2)/(x^2+x-2) dx, you can decompose it into:
(A/(x-1)) + (B/(x+2))
Then solve for A and B, and integrate each term separately. Voilà!
Definite Integrals Explained
Now let’s talk about definite integrals. Unlike indefinite integrals, these give you a specific value instead of a family of functions. The process is pretty straightforward:
1. Find the antiderivative (just like with indefinite integrals).
2. Plug in the upper and lower limits of integration.
3. Subtract the value at the lower limit from the value at the upper limit.
For example, if you’re calculating ∫(from 0 to 1) x^2 dx, the process looks like this:
Antiderivative: (x^3)/3
Plug in limits: [(1^3)/3] - [(0^3)/3]
Result: 1/3
Easy peasy, right?
Applications of Definite Integrals
Definite integrals aren’t just theoretical—they have tons of practical applications. Here are a few examples:
- Area Under a Curve: Definite integrals help you calculate the area between a curve and the x-axis.
- Volume of Solids: You can use integrals to find the volume of 3D shapes like spheres and cones.
- Physics Problems: Integrals are essential for solving problems involving velocity, acceleration, and force.
So yeah, they’re pretty important.
Trigonometric Integrals
Trigonometric functions can make integrals a little more challenging, but don’t worry—we’ve got you covered. Here are some key formulas to keep in mind:
- ∫sin(x) dx = -cos(x) + C
- ∫cos(x) dx = sin(x) + C
- ∫sec^2(x) dx = tan(x) + C
- ∫csc^2(x) dx = -cot(x) + C
These are just the basics, though. For more complex trigonometric integrals, you might need to use techniques like substitution or integration by parts.
Common Trigonometric Identities
Here are a few trigonometric identities that’ll come in handy:
- sin^2(x) + cos^2(x) = 1
- 1 + tan^2(x) = sec^2(x)
- 1 + cot^2(x) = csc^2(x)
These identities can help simplify trigonometric expressions and make integration a lot easier.
Exponential and Logarithmic Integrals
Exponential and logarithmic functions are another common type of integrals. Here are the key formulas:
- ∫e^x dx = e^x + C
- ∫a^x dx = (a^x/ln(a)) + C
- ∫ln(x) dx = xln(x) - x + C
These formulas might look intimidating at first, but with a little practice, they’ll become second nature.
Tips for Solving Exponential Integrals
Here are a few tips to help you tackle exponential integrals:
- Substitution: Use substitution to simplify complex exponential functions.
- Logarithmic Differentiation: Sometimes it’s easier to differentiate first, then integrate.
- Practice, Practice, Practice: The more problems you solve, the better you’ll get.
And remember—don’t be afraid to ask for help if you’re stuck!
Common Mistakes to Avoid
Before we wrap up, let’s talk about some common mistakes people make when working with integrals:
- Forgetting the "+ C": Always include the constant of integration for indefinite integrals.
- Incorrect Substitution: Double-check your substitution variables to make sure they’re correct.
- Misapplying Formulas: Make sure you’re using the right formula for the function you’re integrating.
Avoiding these mistakes will save you a lot of headaches in the long run.
How to Stay Organized
Here are a few tips to help you stay organized while working with integrals:
- Use a Cheat Sheet: Keep your integral cheat sheet handy for quick reference.
- Write Neatly: Clear handwriting makes it easier to spot mistakes.
- Check Your Work: Always double-check your calculations to ensure accuracy.
Staying organized is key to mastering integrals, so don’t skip these steps!
Conclusion
And there you have it—your ultimate guide to mastering integrals with an integral cheat sheet. From basic rules to advanced techniques, we’ve covered everything you need to know to conquer calculus.
Remember, practice makes perfect. The more problems you solve, the more confident you’ll become. And don’t forget to use your cheat sheet—it’s your secret weapon in the world of calculus.
So what are you waiting for? Grab your cheat sheet, sharpen your pencil, and get ready to ace those integrals. And if you found this guide helpful, don’t forget to share it with your friends. Happy calculating!
Table of Contents
Why Do You Need an Integral Cheat Sheet?
Advanced Techniques for Integration
Exponential and Logarithmic Integrals